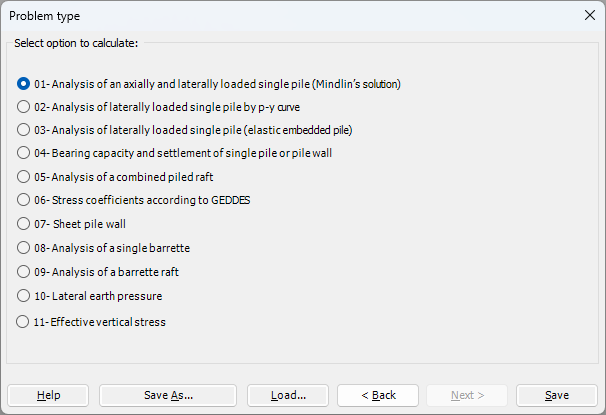
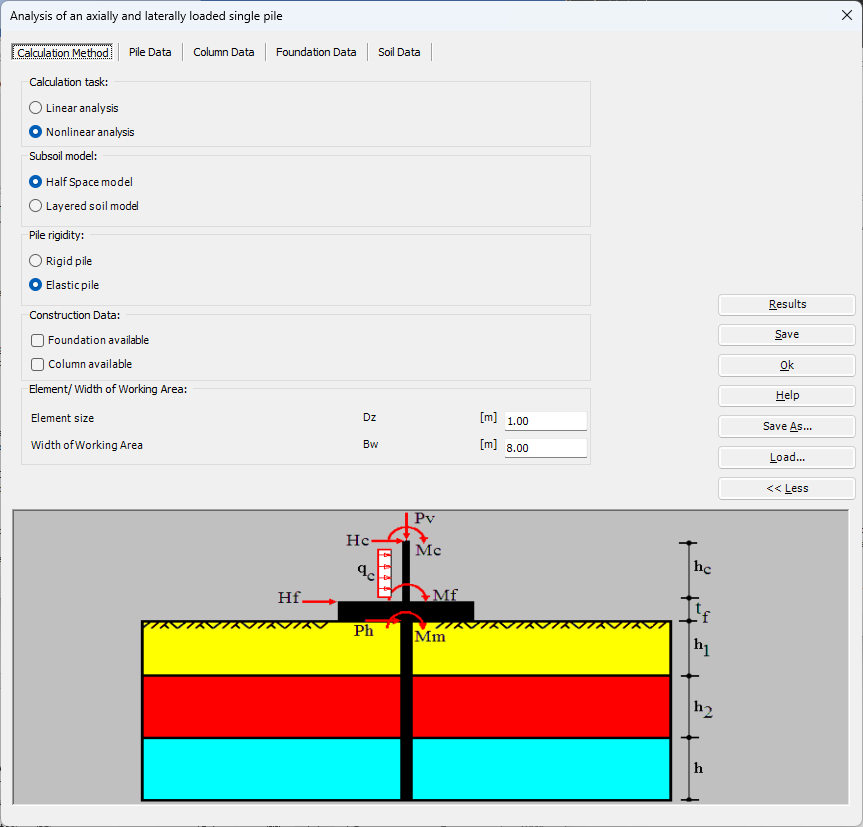
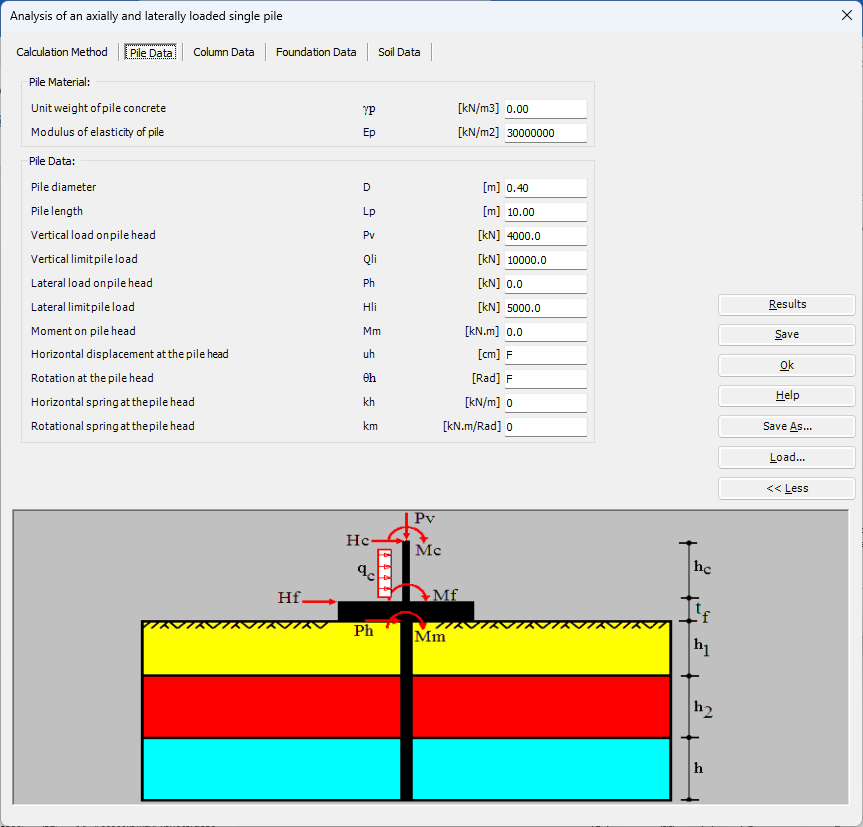
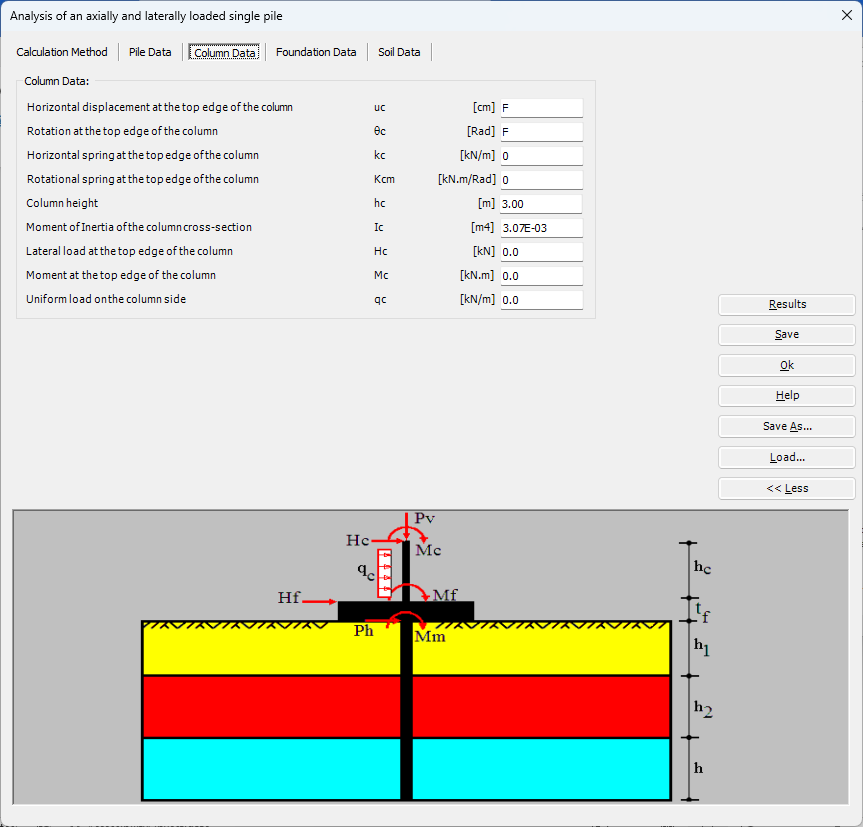
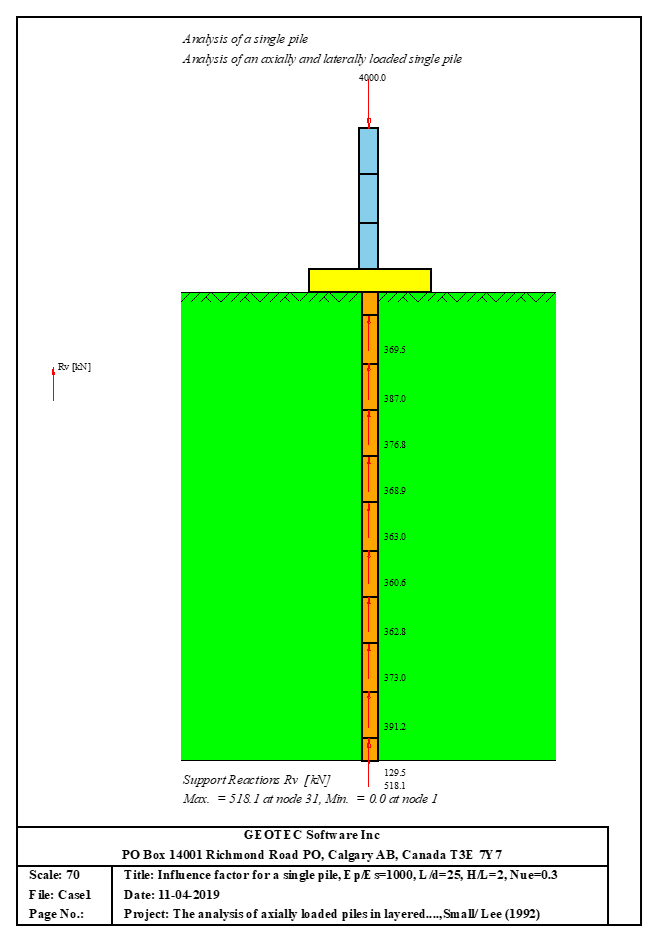
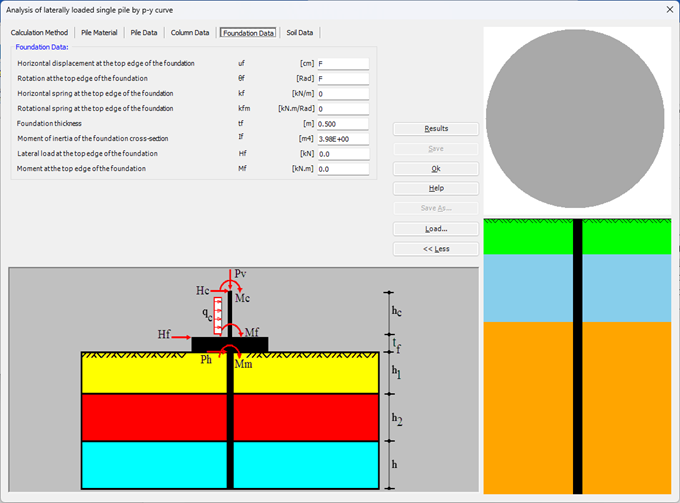
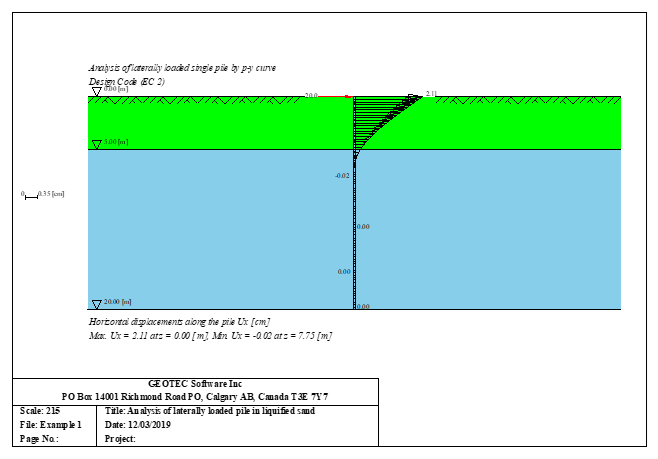
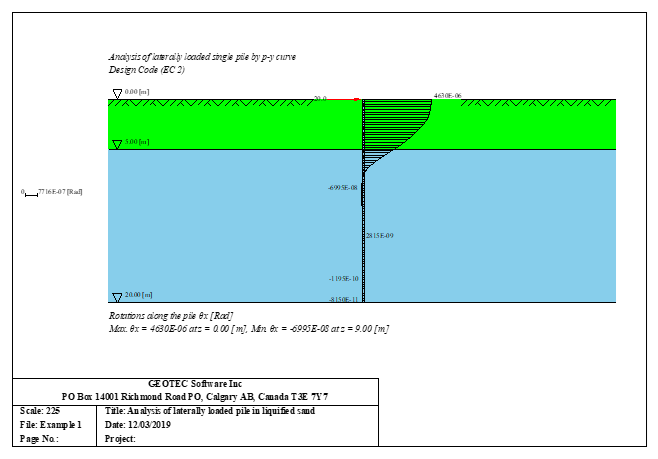
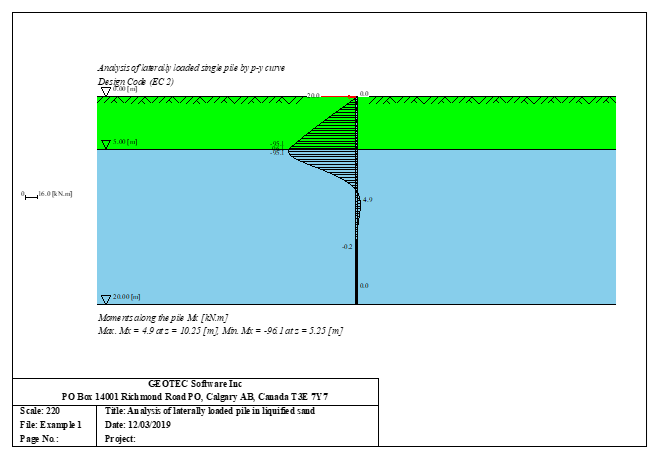
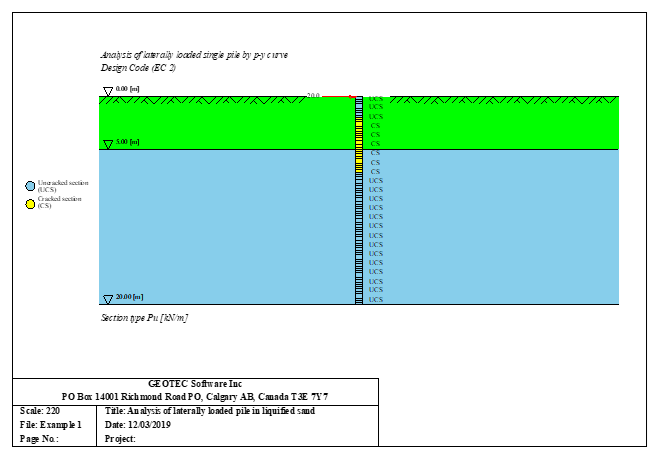
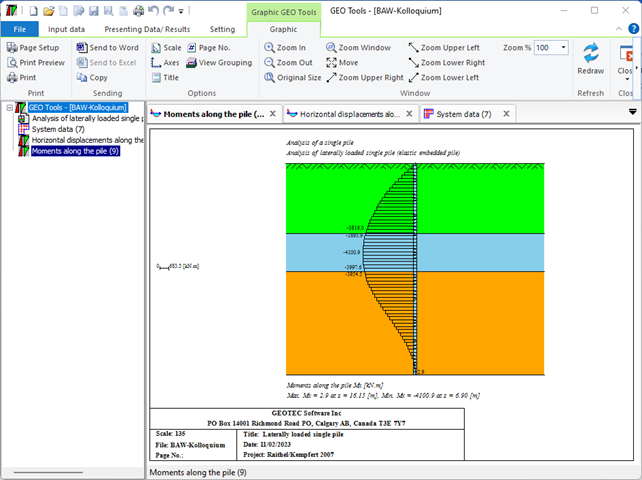
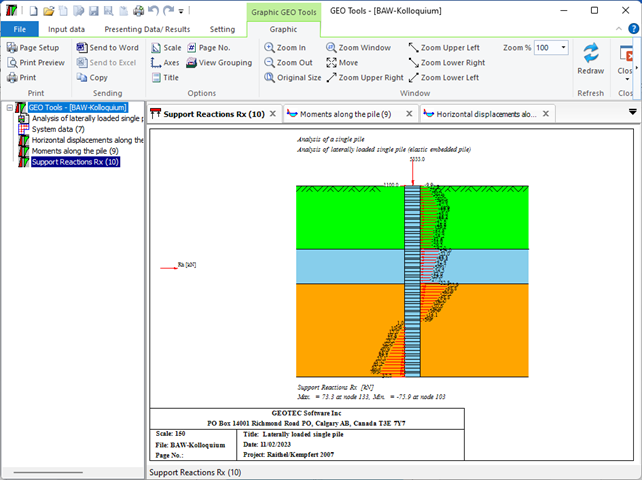
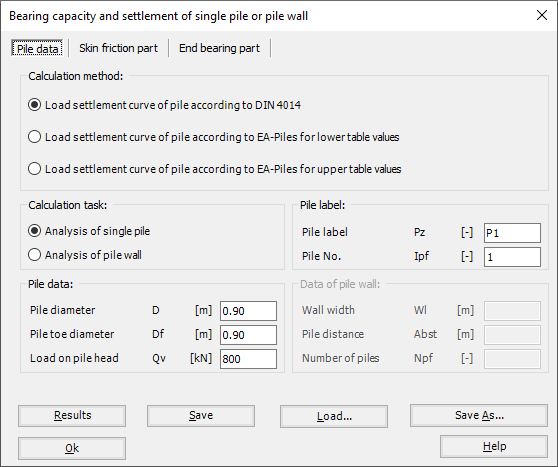
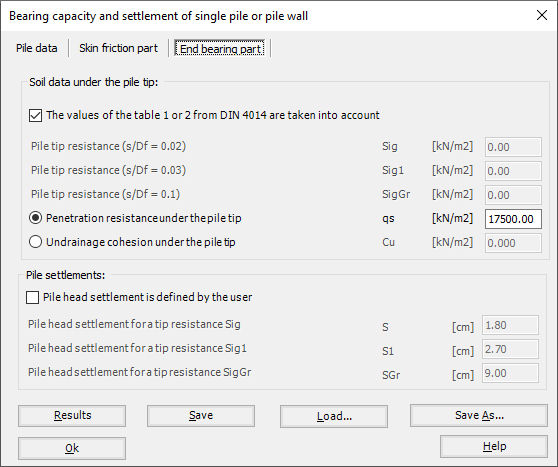
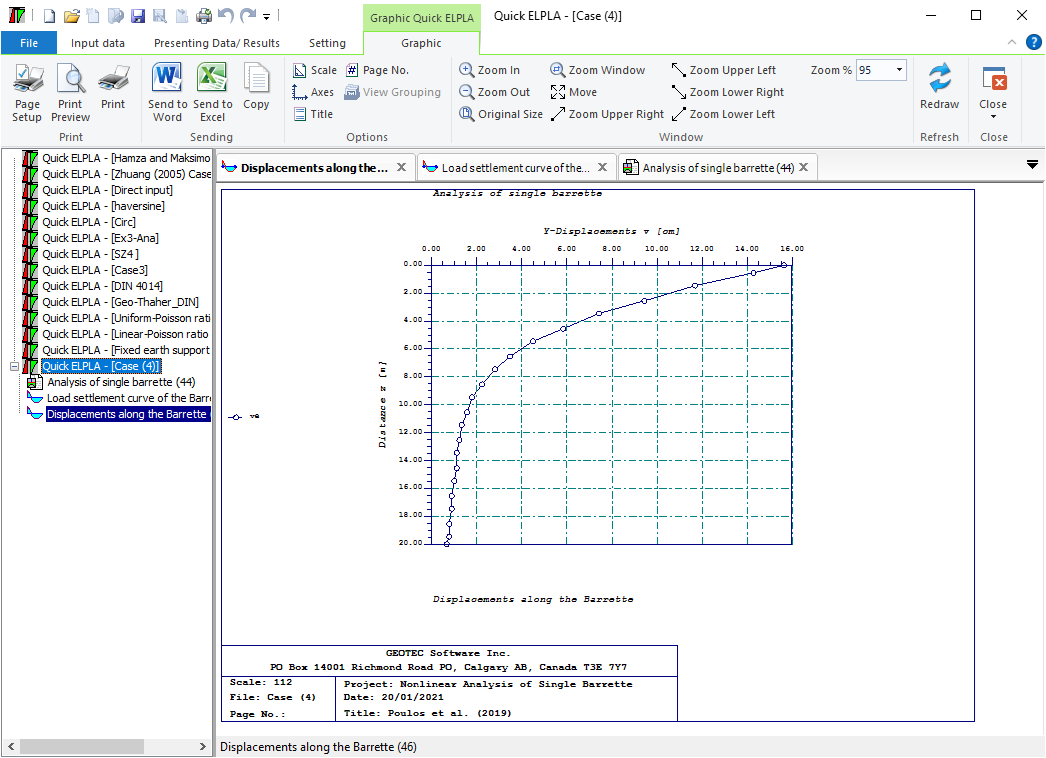
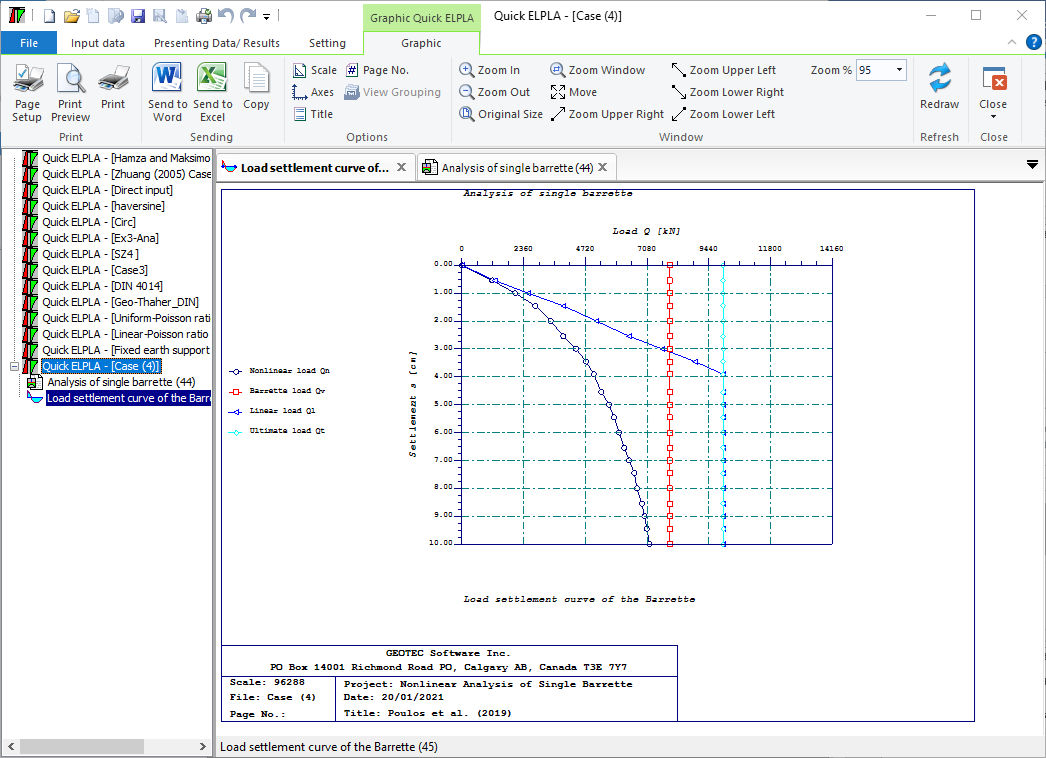
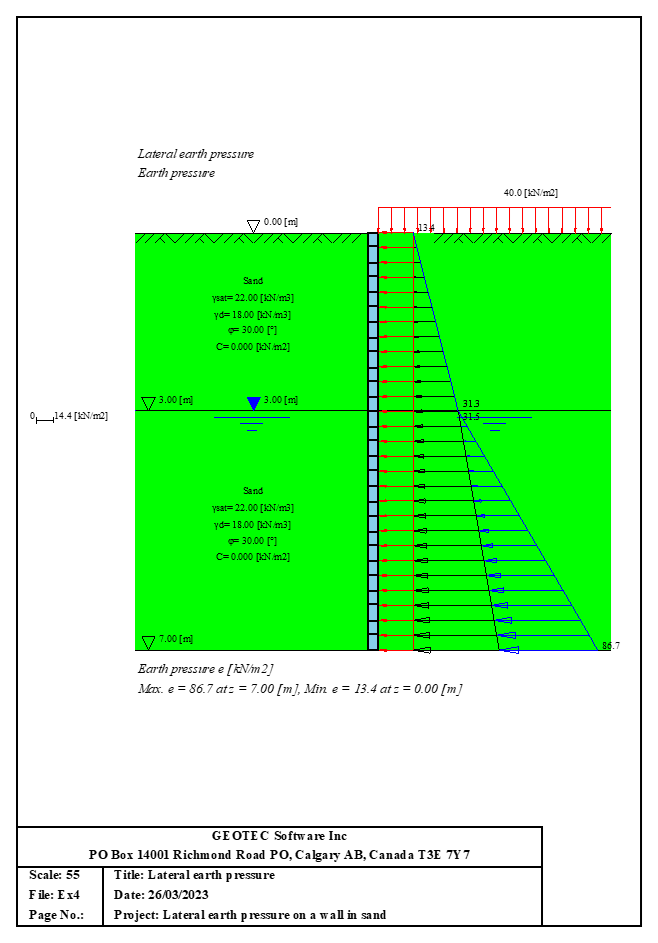
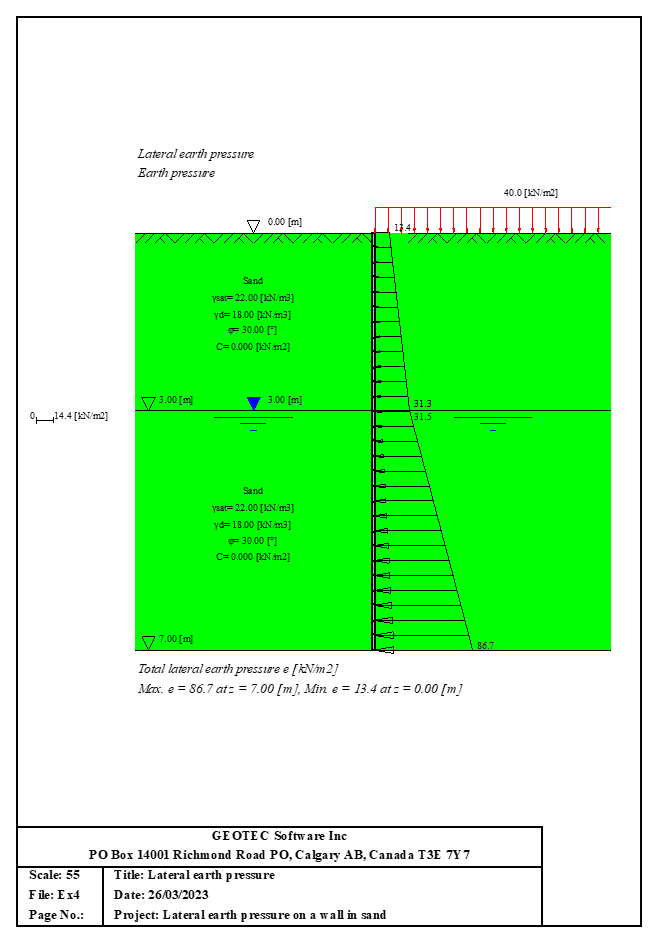
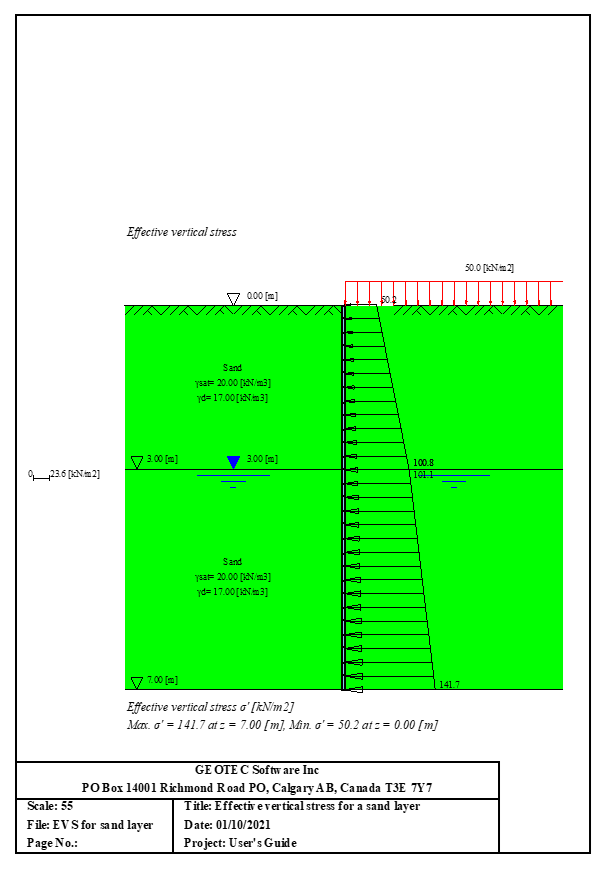
Sheet pile walls can be categorized into anchored or cantilever types and can be analyzed in three ways: as a cantilever, free earth support, or fixed earth support. The Limit Equilibrium Analysis (LEA) method is used to analyze and determine the sheet pile length while the finite element method is used to determine deformations of the sheet pile.
The Limit Equilibrium Analysis (LEA) method is a commonly used technique for analyzing the stability of sheet piles. This method involves evaluating the forces and moments acting on the sheet pile and determining whether the pile is stable under the given loading conditions. The LEA method considers the forces acting on the sheet pile in both the horizontal and vertical directions. The horizontal forces include the soil pressure and any external loads acting on the structure, while the vertical forces include the weight of the pile and any soil or water above the pile. To perform a LEA analysis for a sheet pile, the following steps are typically followed:
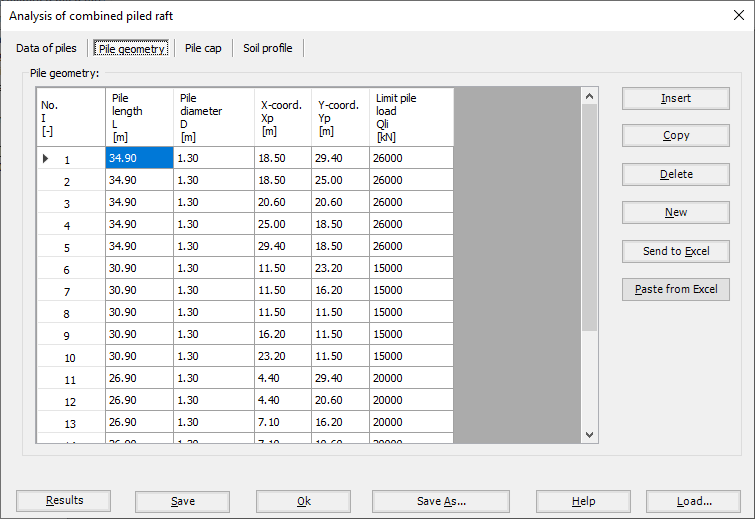
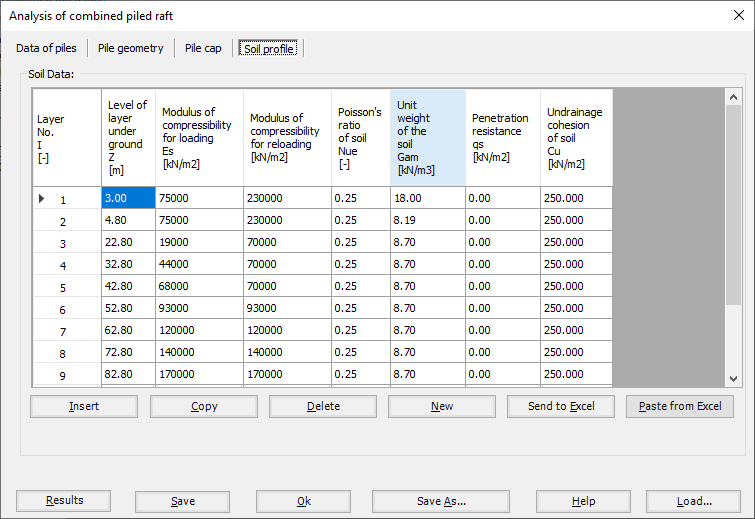
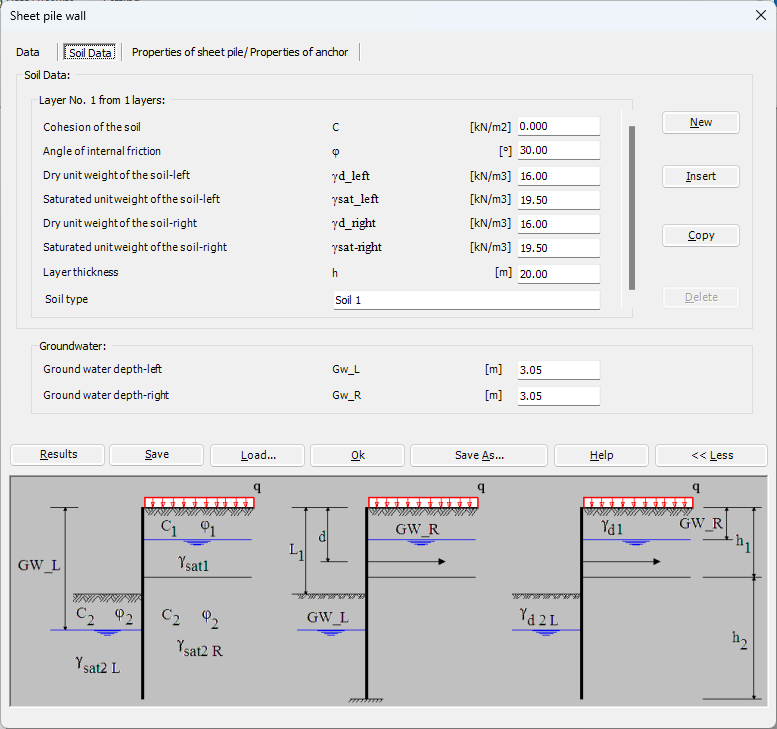
1- Determine the soil properties: The first step in the analysis is to determine the soil properties, including the soil type, strength, and stiffness. This information is used to calculate the soil pressure acting on the sheet pile.
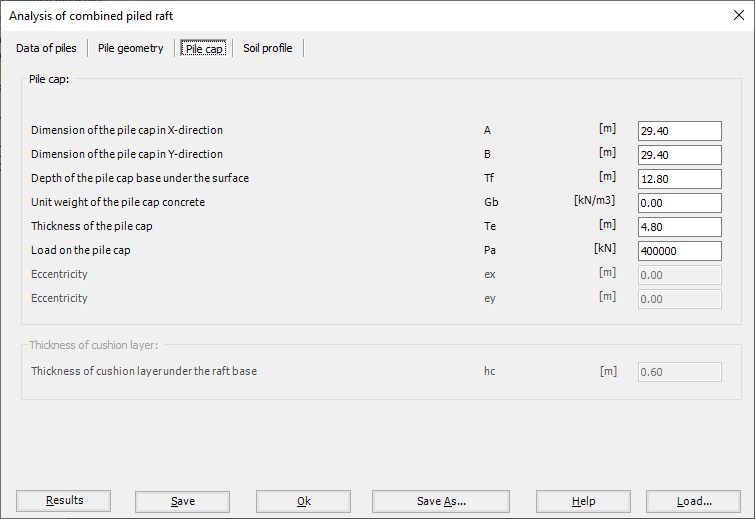
2- Define the loading conditions: The next step is to define the loading conditions, including any external loads and the water level. This information is used to calculate the forces and moments acting on the sheet pile.
3- Calculate the forces and moments: Using the soil properties and loading conditions, the forces and moments acting on the sheet pile are calculated. This includes the soil pressure, water pressure, and any external loads.
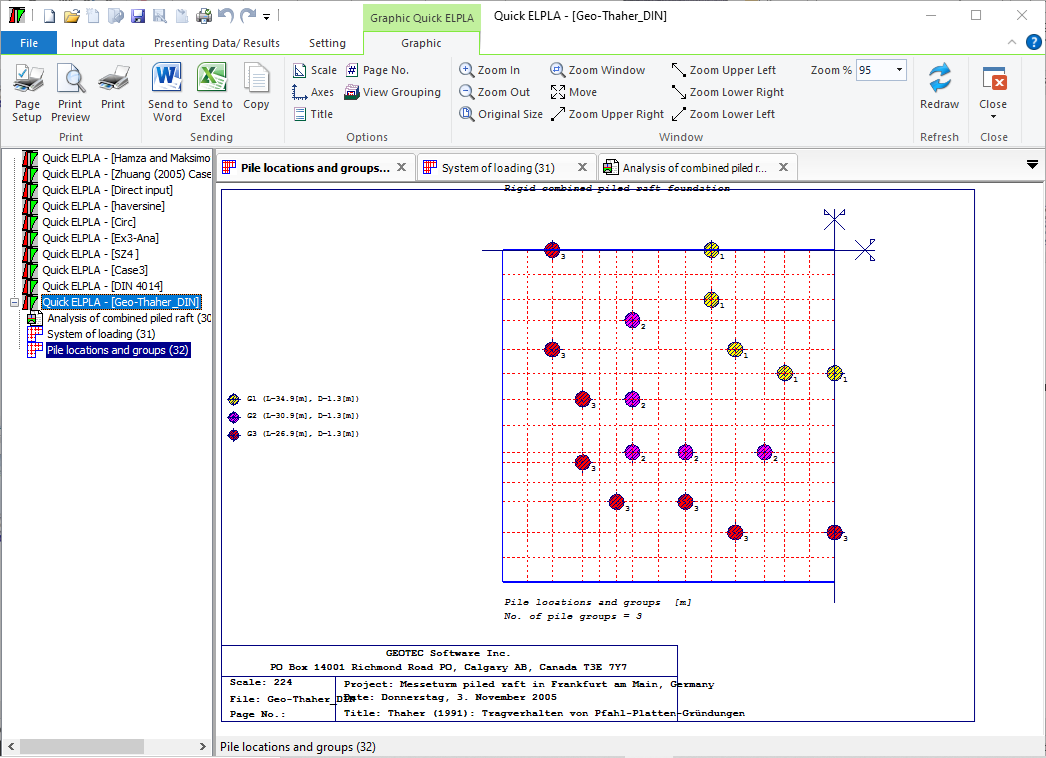
4- Evaluate stability: The final step is to evaluate the stability of the sheet pile. This is done by comparing the forces and moments acting on the pile to its capacity to resist those loads. If the pile is stable, the analysis is complete. If not, modifications to the design may be necessary.
LEA is a widely used method for analyzing the stability of sheet piles, and it can be used to evaluate the performance of different sheet pile configurations and installation methods. However, it is important to note that the results of the analysis are only as accurate as the input data, and careful consideration must be given to the assumptions and limitations of the method.
The Limit Equilibrium Analysis (LEA) method is commonly used to determine sheet pile length and stability, considering forces and moments acting on the sheet pile in both horizontal and vertical directions. The analysis involves determining the soil properties, loading conditions, calculating forces and moments, and evaluating stability. However, the accuracy of the results depends on the input data, and assumptions and limitations of the method must be considered. These are different types of sheet pile walls used in the field of geotechnical engineering and construction five of these can be used in GEO Tools:
1- Cantilever sheet pile wall: This is a type of retaining wall made up of interlocking steel, concrete or wood sheet piles that are driven into the ground. The piles are designed to act as cantilevers, resisting the lateral pressure of soil and water on one side, while being supported by the soil on the other side.
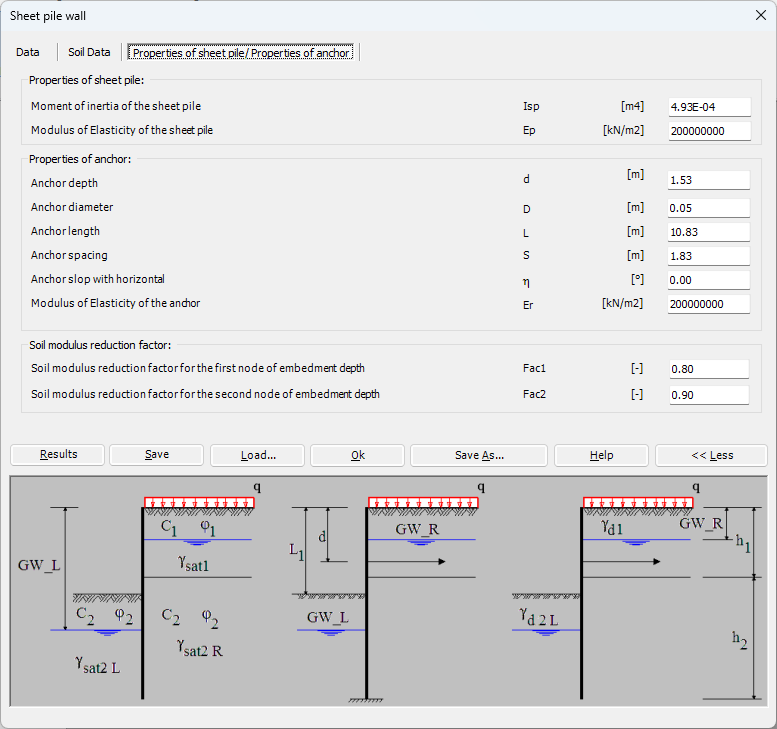
2- Anchored sheet pile wall with free earth support: This type of retaining wall is similar to the cantilever sheet pile wall, but it includes additional support in the form of anchors that are installed into the soil behind the wall. The anchors provide additional resistance against the lateral pressure of the soil and water.
3- Anchored sheet pile wall with fixed earth support (Blum's Method): In this type of retaining wall, the sheet piles are anchored to a rigid structural element such as a concrete beam or slab. The structural element acts as a fixed support, preventing the sheet piles from deflecting inward.
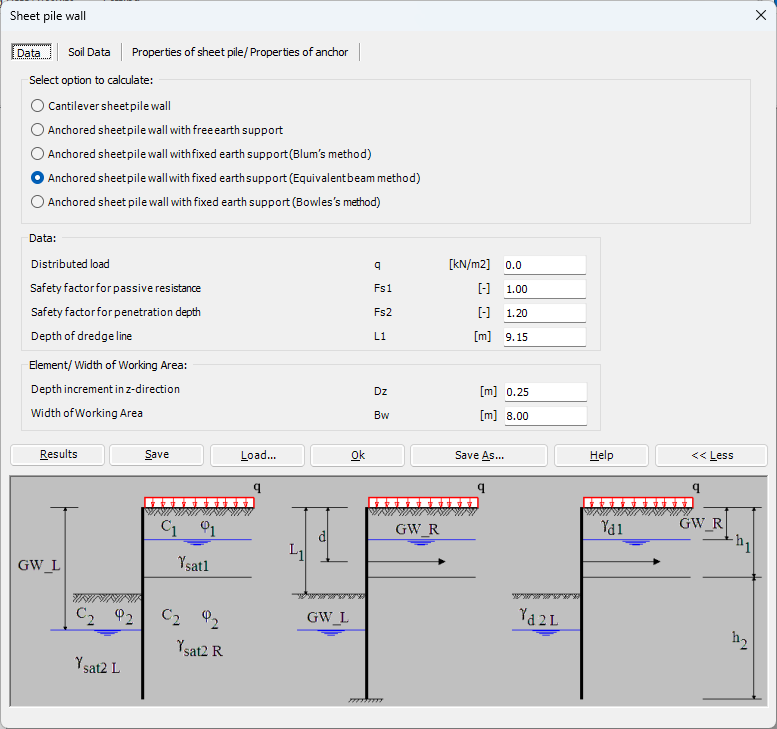
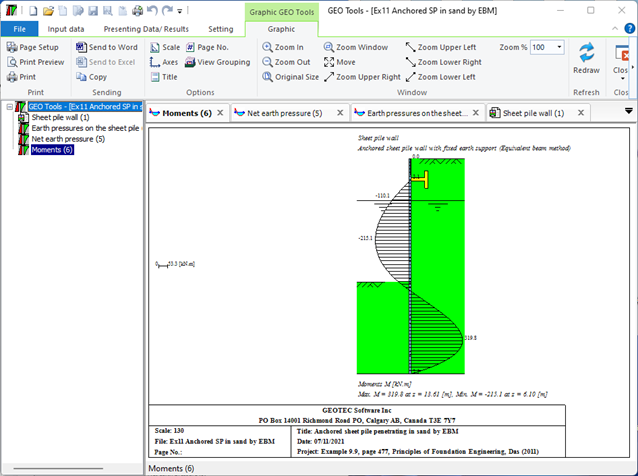
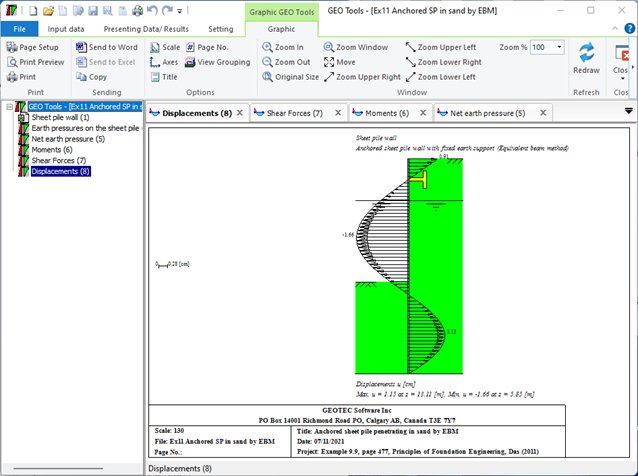
4- Anchored sheet pile wall with fixed earth support (Equivalent beam Method): This method involves treating the sheet pile wall as an equivalent beam and analyzing the forces acting on it. The fixed end of the beam is anchored to a rigid structure, while the free end is supported by the soil. The analysis is used to determine the required size and spacing of the anchors.
5- Anchored sheet pile wall with fixed earth support (Bowels's beam Method): This method is similar to the equivalent beam method, but it also takes into account the soil resistance acting on the sheet pile wall. The analysis involves calculating the bending moment and shear forces acting on the sheet piles and designing the anchor system accordingly.